METHODOLOGY | Teacher Research

DATA COLLECTION

PARTICIPANTS

For the purpose of my research, I invited all elementary teachers from my cyber school to participate. I also invited three local brick-and-mortar teachers to participate. I emailed the teachers a brief description of the research project and attached the signed consent form. All teachers who returned a signed consent form participated in the study. Four cyber teachers and three brick-and-mortar teachers returned a consent form, with a total of seven participants.

DATA COLLECTION PERIOD

Program Evaluation

1. During this time I will look for examples of math intervention models. I will use this information to begin drafting new models of math intervention for cyber schools.

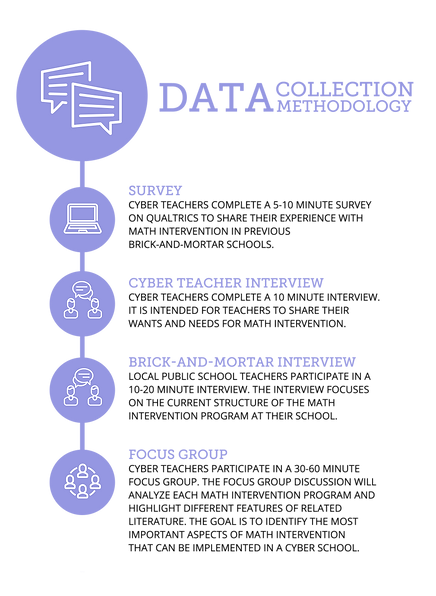
Survey

1. Surveys will be sent by Qualtrics to all cyber school participants who have returned an informed consent document. Surveys will take 5-10 minutes to complete and responses will be requested within five days.

2. Survey data will be collected electronically without identifying information.

Brick-and-Mortar Teacher Interview

1. Interviews will be scheduled by email for each participant. Interviews will be conducted upon IRB approval and no later than March 31, 2020. Interviews will take place by Zoom, at a preferred date and time selected by participants.

2. Interviews will be audio recorded, with participant permission. Interview recording will be deleted after review and before July 1, 2020. Interviews will take 10-20 minutes.

Cyber Teacher Interview

1. Interviews will be scheduled by email for each participant. Interviews will be conducted upon IRB approval and no later than March 31, 2020. Interviews will take place in my Zoom room.

2. Interviews will be recorded, with participant permission. Interview recording will be deleted after review and before July 1, 2020. Interviews will take 10-20 minutes.

Focus Group Discussion

1. Focus group will be convened to review and discuss the models.

2. Focus group participants will be selected from the original set of cyber teacher participants. Selection will include 3-6 teachers who are available to participate in a focus group.

3. Focus group will take place in my Zoom room.

4. The focus group will be audio recorded, with participants’ permission. Focus group recording will be deleted after review and before July 1, 2020. Focus groups will take 45-60 minutes.

LIMITATIONS

One possible limitation is participation from cyber teachers. I hope that a variety of teachers from different grade levels with unique experiences from previous brick-and-mortar settings participate. I can only collect data and information from teachers that return signed consent forms. Another possible limitation is lack of literature on successful math intervention strategies and programs.

LITERATURE

Prior to my research I assumed I was going to discover a universal math intervention model with guidelines for frequency and instruction. However, the literature proves there are multiple models of intervention that support students with math difficulties. I used the literature to identify key components of a successful math intervention program based on commonalities within each article. Overall, the literature suggests that intervention be provided to a diverse group of students. Specifically, in the article “Response to Intervention in Mathematics: Critical Elements,” Lembke, Hampton, and Beyers (2012) share a tiered RTI model:

Tier 1 intervention includes differentiated instruction and flexible grouping in the regular education classroom.
Tier 2 intervention happens in a small group setting outside of the classroom.
Tier 3 intervention is more intensive one on one support.

The majority of the literature recommends that math intervention take place in a small group or a one on one setting (Bryant et al., 2008; Dennis et al., 2016; Flores, Hinton, Burton, 2016; Flores, Hinton, 2019; Gervasoni, 2003; Jitendra et al., 2013; Ketterlin-Geller, Chard, Hank Fien, 2008; Lembke, Hampton, Beyers, 2012; Schumacher, Zumeta Edmonds, Arden, 2017). The frequency at which students participate in intervention varied by article. I compared each article’s frequency and concluded that students with math difficulties require math intervention three to five days a week (Bryant et al., 2008; Flores, Hinton, Burton, 2016; Flores, Hinton, 2019; Jitendra et al., 2013; Ketterlin-Geller, Chard, Hank Fien, 2008; Lembke, Hampton, Beyers, 2012). The literature features effective strategies for math intervention including assessments, instructional strategies, and progress monitoring. Each institution follows a unique guideline to identify students with math difficulties using diagnostics, benchmarks, state assessments, and/or pretests (Bryant et al., 2008; Flores, Hinton, Burton, 2016; Flores, Hinton, 2019; Jitendra et al., 2013; Ketterlin-Geller, Chard, Hank Fien, 2008; Lembke, Hampton, Beyers, 2012). Math interventionists use explicit instruction, Concrete-Representational-Abstract and Schema Based Instruction to support student learning (Baker, Gersten, Dae-Sik Lee., 2002; Bryant et al., 2008; Dennis et al., 2016; Flores,Hinton, Burton, 2016; Flores, Hinton, 2019; Jitendra et al., 2008; Ketterlin-Geller, Chard, Hank Fien, 2008; Lembke, Hampton,Beyers, 2012). Lastly, the math interventionist monitors student progress to influence instruction.

TEMPLATES

The literature helped me find key components of successful math intervention models. I used the literature to identify themes within math intervention groups and effective strategies. I categorized the qualitative data from surveys, interviews, and the focus group using the themes found in the literature. Finally, the themes in the literature were used to organize my findings and conclusions.

DATA ANALYSIS PROCESS

I found themes within the literature that support successful math intervention programs and models. I organized the literature by group (size, frequency, ability) and effective strategies (assessment, instruction, progress monitoring). I used the themes found in the literature and applied them to the survey, interviews, and focus group responses. I read the survey responses and transcribed each interview. Then I connected the experiences of the cyber and brick-and-mortar teachers to the literature. I recorded and listened to the focus group and noted each teacher’s comments about restructuring the current model of intervention to better suit the needs of their students and related their comments to the emergent themes.

REFLECTION

The literature supporting math intervention has several factors to consider within each model. I met with Dr. Leaman to discuss my findings from the literature. With Dr. Leaman’s support I was able to name a theme: key components of successful math intervention models. After reading through the literature and interviewing brick-and-mortar teachers I realized that there is more than one right answer on how to best support students with math difficulties. Therefore, I decided it was best to use the brick-and-mortar models and bullet points from the literature during the focus group rather than creating a variety of math intervention models on my own. This way, participants were able to discuss key components from existing math intervention models to incorporate into our cyber school setting.